On this page, I describe how the chemical formulas are written, how to obtain them and I finish with a set of solved exercises for practice.
The outline of this chapter is:
- Atomic theory.
- Atomic masses and moles.
- Molecules and molecular mass.
- Percentage composition
- Empirical formulas.
- Molecular formulas.
- Exercises.
- Comments
Atomic theory
During the 19th century, the atomic theory developed. At the beginning of the century, Dalton proposed that molecules are formed by the combination of atoms. The idea was developed over the century. The main points of Dalton’s atomic theory, as it eventually developed, are:
- Elements are made of extremely small particles called atoms.
- Atoms of a given element have similar mass and properties, atoms of different elements differ in mass and properties.
- Atoms of different elements combine in simple whole-number ratios to form chemical compounds.
- In chemical reactions, atoms are combined, separated or rearranged, but not destroyed.
Atomic masses and moles
Note: You will find that sometimes in chemistry, mass(es) and weight(s) are use interchangeably. Both terms mean the same for a chemist, however, a physic will disagree. I will use the term mass or masses.
As the atomic theory was developing, the next step was to determine the masses of atoms. As the atoms are so small, they could not be measured at the time that the theory was developed and therefore it was decided to refer to a relative scale of masses between atoms and defined a new unit: the atomic mass unit (amu). Initially, it was developed using as a unit the mass of the hydrogen, however, over time it was changed to use the most common element found, that is isotope of carbon 12 (or 12C) and therefore:
1 atom of 12C = 12 amu
Based on this, the values of atomic masses were developed and are given in the periodic table.
As chemical reactions involved a considerable number of atoms, the use of amu was not very practical in real terms, therefore it was defined a new unit: the mole (abbreviated as mol). A mole is defined as the number of atoms present in 12 g of 12C. As science developed, it was possible to quantify the numbers of atoms in 12 g of 12C, this number is 6.0221 × 1023 and is known as Avogadro’s number (NA).
Avogadro’s number (NA) = 6.0221 × 1023 is the number of particles in 1 mol.
In practical terms, when we are working with atomic masses, you can use amu or g/mol.
Molecules and molecular mass
Following the atomic theory, we can define chemical species based on the atoms that constitute the compound. The nomenclature is to write the elements that constitute the molecules, indicating with a subindex the number of atoms, for example, water has the formula H2O, meaning that it is formed by 2 atoms of hydrogen (H) and 1 atom of oxygen (O).
In nature, we have discrete molecules where the atoms are bonded by covalent bonds (like the water that we mentioned above) and other compounds where the atoms are bonded by ionic bonds, forming larger crystalline structures, like the NaCl (salt that we use in food).
This is applicable when we have discrete molecules formed by covalent bonds (like water). But there are some compounds that are not formed by single molecules, they are formed by ions than tend to form large structures, in this case, they are called ‘formula units’ and it is the simplest formulation that we can have for this compound. For example, the salt that we use in the food, is formed by crystals containing the same number of atoms of sodium (Na) and chlorine (Cl). The ‘formula unit’ of this salt is NaCl. Sometimes, formula units are referred as molecules as well, it is not technically correct, but it is a common practice.
A compound’s molecular mass (Mr, however sometimes referred to as Mw) is the sum of the atomic masses of all the atoms that appear in its formula. For example, if the atomic mass of the hydrogen is 1 g/mol and the atomic mass of oxygen is 16 g/mol, the molecular mass of the water (H2O) is:
Molecular mass of H2O =
= 2 x atomic mass of H + (1 x) atomic mass of O =
= 2 x 1 + 16 = 18 g/mol
The relationships between mass, molecular weight and the number of moles are:

You can summarize it in the following triangle equation:
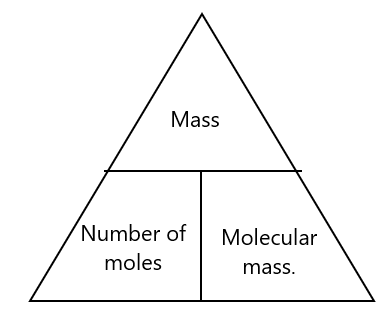
Example 1.
The molecular formula of sulfuric acid is H2SO4. a) What is it molecular mass? b) How many moles are in 500 g of H2SO4? c) What is the mass of 2.5 moles of H2SO4? The respective atomic masses are: H: 1.008 g/mol, S: 32.065 g/mol and O: 15.999 g/mol. (You can round these atomic masses to 1, 32 and 16 respectively, however, I will use 3 decimal places to minimize rounding errors).
Answer
The molecular mass of the H2SO4 is
2 x atomic mass of H + 1 x atomic mass of S + 4 x atomic mass of O =
= 2 x 1.008 + 1 x 32.065 + 4 x 15.999 =
= 2.016 + 32.065 + 63.996 =
= 98.077 g/mol.
To find the number of moles in 500 g of sulfuric acid, you can use proportions:

or if you prefer to work with units:
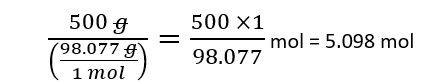
To calculate how much weight 2.5 moles of H2SO4, simply multiply the molecular mass by the number of moles:
2.5 mol x 98.077 g/mol = 245.193 g (rounded to 3 decimal places)
Note: When using molecular weights, there is a rounding error depending on how many decimal places you use. It will be common to have similar numbers, but not the same. For example, if instead of using 98.077 g/mol, I use 98.1 g/mol, I get that 2.5 moles weight 245.250 g (not 245.193 g). In most cases, it will not make too much difference (if accuracy is needed, the chemist has tools to achieve this accuracy at his/her disposal). For our purpose here, if you repeat the calculations by yourself or find tabulated values that are similar to those that I have, we can consider that we have the same result.
Example 2
If the atomic mass of the sodium (Na) is 22.990 g/mol and the chlorine (Cl) is 35.453 g/mol:
a) What is the molecular mass of sodium chloride (NaCl)?
b) How many moles do we have in 200 g of NaCl?
c) What is the weight of 4.5 moles of NaCl?
Answers
a) The molecular is:
1 x atomic mass of Na + 1 x atomic mass of Cl =
= 1 x 22.990 + 1 x 35.453 =
= 58.443 g/mol
b) As the molecular mass is 58.443 g/mol, 200 g are:
200 g / 58.443 g/mol= 3.422 mol
c) As the molecular mass is 58.443 g/mol, 4.5 moles are:
4.5 mol x 58.443 g/mol = 262.994 g
Example 3
If the atomic masses of the potassium (K) is 39.098 g/mol, the manganese (Mn) is 54.938 g/mol and the oxygen (O) is 15.999 g/mol:
a) what is the molecular mass of potassium permanganate (KMnO4)?
b) How many moles do we have in 450 g of KMnO4?
c) What is the weight of 1.5 moles of KMnO4?
Answer
a) The molecular mass is:
1 x atomic mass of K + 1 x atomic mass of Mn + 4 x atomic mass of O =
= 1 x 39.083 + 1 x 54.938 + 4 x 15.999 =
= 1 x 39.083 + 1 x 54.938 + 63.996 =
= 158.032 g/mol
b) As the molecular mass is 158.032 g/mol, 450 g are:
450 g / 158.032 g/mol = 2.848 moles
c) As the molecular mass is 158.032 g/mol, 1.5 moles are:
1.5 moles x 158.032 g/mol = 237.048 g
Percentage composition
The percentage that each element contributes to the total weight of a compound is calculated by the formula:
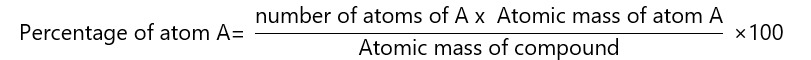
When the number of the atoms is 1, it is not usually written.
Example
What is the percentage of potassium (K), manganese (Mn) and oxygen (O) in the potassium permanganate (KMnO4)? Atomic masses K: 39.098 g/mol, Mn: 54.938 g/mol, O = 15.999 g/mol.
The molecular mass of the potassium permanganate (KMnO4) is:
1 x atomic mass of K + 1 x atomic mass of Mn + 4 x atomic mass of O =
= 1 x 39.083 + 1 x 54.938 + 4 x 15.999 =
= 1 x 39.083 + 1 x 54.938 + 63.996 =
= 158.032 g/mol
The different percentages is:
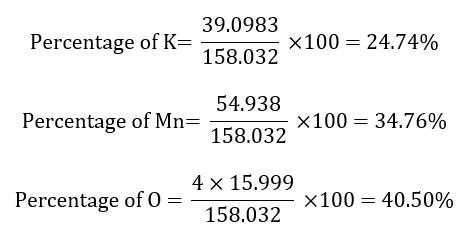
Adding all the percentages, we obtain 100%: 24.74 + 34.76 + 40.50 = 100.00. Sometimes, we get that the answer is 99.99% instead of 100.00%. Is it wrong? No, it is due to rounding errors and we can consider that the answer is correct.
Empirical formulas
Sometimes, we only know the masses or percentages of the elements in a chemical compound. The process for finding the formula is the same in both cases, as we assume the total mass is 100 grams (or any other unit).
Steps to find the formula:
- Find the number of moles for each element:
Divide the mass (or percentage) of each element by its atomic mass. This gives the moles of each element.
Tip: Use at least 2 decimal places to avoid rounding errors that could give the wrong answer. - Determine the simplest ratio:
Divide the moles of each element by the smallest number of moles found. This gives the ratio of the elements in the compound. - Adjust to whole numbers:
If the ratios are not whole numbers, multiply them by the same factor to make them whole.
It might be helpful to create a table to develop the problem with the following headings:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| … | … | … | … | … |
| … | … | … | … | … |
(see the samples below to see how to fill it).
Example 1.
What is the empirical formula of a component that contains 60 g of sulfur and 90 g of oxygen? Atomic masses: S = 32.066 g/mol, O = 15.999 g/mol
Answer
1. We first divide the weight of each element found by its atomic mass:
% S: 60 g / 32.066 g/mol = 1.871 mol
% O: 90 g / 15.999 g/mol = 5.621 mol
2. The lower number of mols is 1.871 for the S, therefore, we divided both by 1.871:
S: 1.871 / 1.871 = 1
O: 5.621 / 1.871 = 3
Or creating a the table:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| S | 32.066 | 60 | 60 / 32.066 = 1.871 | 1.871/1.871 = 1 |
| O | 15.999 | 90 | 90/15.999 = 5.625 | 5.625/1.871 = 3 |
As the ratio is 3 moles of O for each mole of S, the empirical formula is SO3.
Example 2.
What is the empirical formula of a component that contains 26.57% of potassium, 35.36% of chromium and 38.07% of oxygen? Atomic masses: K = 39.098 g/mol, Cr = 51.996, O = 15.999 g/mol.
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| K | 39.098 | 26.57 | 26.57 / 39.098 = 0.680 | 0.680 / 0.680 = 1 |
| Cr | 51.996 | 35.36 | 35.36 / 51.996 = 0.680 | 0.680 / 0.680 = 1 |
| O | 15.999 | 38.07 | 38.07 / 15.999 = 2.380 | 2.380 / 0.680 = 3.5 |
This would give us the formula: KCrO3.5, however, as the formulas are expressed in whole numbers, we multiply by 2 the contribution of each atom to obtain: K2Cr2O7, (potassium dichromate).
Molecular formulas
Empirical formulas give the proportion of atoms in molecules, however, there are molecules that can have the same proportions of atoms. For example, the methane (CH4) and the ethene (C2H8), both have the same proportion of atoms (4 atoms of hydrogen for each atom of hydrogen) but they have different number of atoms (5 in the methane and 10 in the ethene). When a formula gives the proportion of atoms and the total number of atoms, it is a molecular formula. To clarify:
- The empirical formula of a compound is the simplest, indicating the ratio of atoms of each element in a compound.
- The molecular formula gives the actual number of atoms of each element present in a compound.
In some cases, the empirical formula and the molecular formula are the same. Examples:
| Empirical formula | Molecular formula |
| H2O | H2O |
| CO2 | CO2 |
| CH4 | CH4, C2H8 |
| NO2 | N2O4 |
| CH3O | C2H6O2 |
| CH2O | C6H12O6 |
How can we find the molecular formula from the empirical formula? It is done by knowing the molecular mass of the compound. There are different ways to determine the molecular mass of compounds, however, I will not cover them here. I will explain how to determine the molecular formula from the empirical formula and the molecular mass of the molecular formula. For example, we have a sample that its empirical formula is CH4 and its molecular mass is 32.086 g/mol. We can consider that the compound has the molecular formula (CH4)n(or CnH4n), where n is:
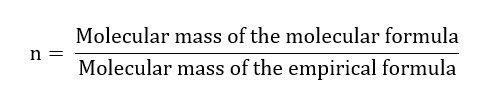
The molecular mass of the empirical formula (CH4) is:
atomic mass of C + 4 x atomic mass of H =
= 12.011 + 4 x 1.008 =
= 16.043 g/mol
As the molecular mass of the molecular formula is 32.086 g/mol,
n = 32.086 / 16.043 = 2
Therefore, the molecular formula is C2H4 x 2 = C2H8.
Example 1
A compound with empiric formula CH2O has a molecular mass of 180.156 g/mol. What is the molecular formula of the compound? Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol.
The molecular mass empirical formula is
atomic mass of C + 2 x atomic mass of H + atomic mass of O =
= 12.011 + 2 x 1.008 + 15.999 =
= 30.026 g/mol
Therefore n = 180.156 / 30.026 = 6 and the molecular formula is (CH2O)n = CnH2nOn = C6H12O6
Example 2
A compound is formed with 92.26% of carbon and 7.74% of hydrogen. Its molecular mass is 78.114 g/mol. Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol.
We start calculating the empirical formula:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 92.26 | 92.26 / 12.011 = 7.681 | 7.681 / 7.679 = 1 |
| H | 1.008 | 7.74 | 7.74 / 1.008 = 7.679 | 7.679 / 7.679 = 1 |
Therefore, the empirical formula is CH, and its molecular mass is:
atomic mass of C + atomic mass of H =
= 12.011 + 1.008 =
= 13.019 g/mol
If we divide the molecular mass of the compound by the molecular mass of the empirical formula:
n = 78.114 / 13.019 = 6
Giving the molecular formula (CH)n = (CH)6 = C6H6
Exercises
Molecular mass
1. Calculate the molecular mass of Na2SO4. Atomic masses: Na: 22.990 g/mol, S: 32.066 g/mol, O: 15.999 g/mol.
Answer
Na: 2 x 22.990 = 45.980
S: 1 x 32.066 = 32.066
O: 4 x 15.999 = 63.996
Na2SO4 = 45,980 + 32.066 + 63.996 = 142.042 g/mol
2. Calculate the molecular mass of (NH4)2SO3. Atomic masses: N: 14.007 g/mol, H: 1.008 g/mol, S: 32.066 g/mol, O: 15.999 g/mol.
Answer
As the NH4 appears twice, in the calculation we should count as N2H8SO3
N: 2 x 14.007 = 28.014
H: 8 x 1.008 = 8,064
S: 1 x 32.066 = 32,066
O: 3 x 15.999 = 47.997
(NH4)2SO3 = 28.014 + 8.064 + 32.066 + 47.997 = 116.141 g/mol
3. Calculate the molecular mass of H3PO4. Atomic masses: H: 1.008 g/mol, P: 30.974 g/mol, O: 15.999 g/mol.
Answer
H: 3 x 1.008 = 3.024
P: 1 x 30.974 = 30.974
O: 4 x 15.999 = 63.996
H3PO4 = 3.024 + 30.974 + 63.995 = 97.994 g/mol
4. Calculate the molecular mass of Hg2(NO3)2. Atomic masses: Hg: 200.59 g/mol, N: 14.007 g/mol, O: 15.999 g/mol.
Answer
As the nitrate ion (NO3–) appears twice, we should count as Hg2N2O6
Hg: 2 x 200.59 = 401.18
N: 2 x 14.007 = 28.014
O: 6 x 15.999 = 95.994
Hg2(NO3)2 = 401.18 + 28.014 + 95.994 = 525.188 g/mol
5. Calculate the molecular mass of Al2(SO3)3. Atomic masses: Al: 26.982 g/mol, S: 32.066 g/mol, O: 15.999 g/mol.
Answer
As the sulfite ion (NO32-) appears three times, we should count as Al2S3O9
Al: 2 x 26.982 = 53.964
S: 3 x 32.066 = 96.198
O: 9 x 15.999 = 143.991
Al2SO3 = 53.964 + 96.198 + 143.991 = 294.153 g/mol.
Moles
1. How many moles of is 100 g of (NH4)2SO3? Atomic masses: N: 14.007 g/mol, H: 1.008 g/mol, S: 32.066 g/mol, O: 15.999 g/mol.
Answer
Mr (NH4)2SO3: 2 x 14.007 + 8 x 1.008 + 1 x 32.066 + 3 x 15.999 = 116.141 g/mol
100 g of (NH4)2SO3 is 100 g / 116.141 g/mol = 0.861 mol
2. How many moles of is 250 g of Na2HPO4? Atomic masses: Na: 22.990 g/mol, H: 1.008 g/mol, P: 30.974 g/mol, O: 15.999 g/mol.
Answer
Mr Na2HPO4: 2 x 22.990 + 1 x 1.008 + 1 x 30.974 + 4 x 15.999 = 141.958 g/mol
250 g of Na2HPO4 is 250 g / 141.958 g/mol = 1.761 mol
3. How many moles of is 400 g of KNO3? Atomic masses: K: 39.098 g/mol, N: 14.007 g/mol, O: 15.999 g/mol
Answer
Mr KNO3: 1 x 39.098 + 1 x 14.007 + 3 x 15.999 = 101.103 g/mol
400 g of KNO3 is 400 g / 101.103 g/mol = 3.956 mol
4. How many moles of is 350 g of Ca(MnO4)2? Atomic masses: Ca: 40.078 g/mol, Mn: 54.938 g/mol, O: 15.999 g/mol
Answer
Mr Ca(MnO4)2: 1x 40.078 + 2 x 54.938 + 8 x 15.999 = 277.946 g/mol
350 g of Ca(MnO4)2 is 350 g / 277.946 g/mol = 1.259 mol
5. How many moles of is 800 g of Fe2(SO4)3. Atomic masses: Fe: 55.933 g/mol, S: 32.066 g/mol, O: 15.999 g/mol
Answer
Mr Fe2(SO4)3: 2 x 55.933 + 3 x 32.066 + 12 x 15.999 = 400.052 g/mol
800 g of Fe2(SO4)3 is 800 g / 400.052 g/mol = 2.000 mol
6. What is the mass of 2 moles of NaHCO3? Atomic masses: Na: 22.990 g/mol, H: 1.008 g/mol, C: 12.011 g/mol, O: 15.999 g/mol
Answer
Mr NHCO3: 1 x 22.990 + 1 x 1.008 + 1 x 12.011 + 3 x 15,999 = 84.006 g/mol
2 moles of NHCO3 are 2 mol x 84.006 g/mol = 168.012 g
7. What is the mass of 2.5 moles of H2SO4? Atomic masses: H: 1.008 g/mol, S: 32.066 g/mol, O: 15.999 g/mol
Answer
Mr H2SO4: 2 x 1.008 + 1 x 32.066 + 4 x 15,999 = 98.078 g/mol
moles of NHCO3 are 2.5 mol x 98.078 g/mol = 245.195 g
8. What is the mass of 1.5 moles of K3PO4? Atomic masses: K: 39.098 g/mol, P: 30.974 g/mol, O: 15.999 g/mol
Answer
Mr K3PO4: 3 x 39.098 + 1 x 30.974 + 4 x 15,999 = 212.264 g/mol
1.5 moles of K3PO4 are 1.5 mol x 212.264 g/mol = 318.395 g
9. What is the mass of 0.5 moles of NaCN? Atomic masses: Na: 22.990 g/mol, C: 12.011 g/mol, N: 14.007 g/mol
Answer
Mr NaCN: 1 x 22.990 + 1 x 12.011 + 1 x 14,007 = 49.008 g/mol
0.5 moles of NaCN are 0.5 mol x 49.008 g/mol = 24.504 g
10. What is the mass of 3.5 moles of HgCl2. Atomic masses: Hg: 200.59 g/mol, Cl: 35.453 g/mol
Answer
Mr HgCl2: 1 x 200.59 + 2 x 35.453 = 271.496 g/mol
3.5 moles of HgCl2 are 3.5 mol x 271.496 g/mol = 950.236 g
Percentage composition
Note: the results of the percentage are rounded to 2 decimal places.
1. Calculate the percentage composition of ammonia (NH3). Atomic masses: N: 14.007 g/mol, H: 1.008 g/mol
Answer
Mr NH3: 1 x 14.007 + 3 x 1.008 = 17.031 g/mol
% N: (14.007 / 17.031) x 100 = 82.24%
% H: (3 x 1.008 / 17.031) x 100 = 17.76%
Check: 82.24 + 17.76 = 100
2. Calculate the percentage composition of Ca3(PO4)2. Atomic masses: Ca: 40.078 g/mol, P: 30.974 g/mol, O: 15.999 g/mol.
Answer
Mr Ca3(PO4)2: 3 x 4.078 + 2 x 30.974 + 8 x 15.999 = 310.174 g/mol
% Ca: (3 x 4.078 / 310.174) x 100 = 38.76%
% P: (2 x 30.974 / 310.174) x 100 = 19.97%
% O: (4 x 15.999 / 310.174) x 100 = 41.26%
Check: 38.76 + 19.97 + 41.26 = 99.99, it is not 100.00 due to rounding errors in the calculations. In these cases, we can consider that the solution given is correct (you can check that the result is correct by using all the decimal places when adding the results).
4. Calculate the percentage composition of butane (C4H10). Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol.
Answer
Mr C4H10: 4 x 12.011 + 10 x 1.008 = 58.124 g/mol
% C: (4 x 12.011 / 58.124) x 100 = 82.66%
% H: (10 x 1.008 / 58.124) x 100 = 17.34%
Check: 82.66 + 17.34 = 100
5. Calculate the percentage composition of magnesium phosphate (Mg3(PO4)2). Atomic masses: Mg: 24.305 g/mol, P: 30.974 g/mol, O: 15.999 g/mol
Answer
Mr Mg3(PO4)2: 3 x 24.306 + 2 x 30.974 + 8 x 15.999 = 262.855 g/mol
% Mg: (3 x 24.306 / 262.855) x 100 = 27.74%
% P: (2 x 30.974 / 262.855) x 100 = 23.57%
% O: (8 x 15.999 / 262.855) x 100 = 48.69%
Check: 27.74 + 23.57 + 48.69 = 100
6. Calculate the percentage composition of silver chromate (Ag2CrO4). Atomic masses: Ag: 107.868 g/mol, Cr: 51.996 g/mol, O: 15.999 g/mol
Answer
Mr Ag2CrO4: 2 x 107.868 + 51.996 + 4 x 15.999 = 331.728 g/mol
% Ag: (2 x 107.868 / 331.728) x 100 = 65.03%
% Cr: (51.996 / 331.728) x 100 = 15.67%%
% O: (4 x 15.999 / 331.728) x 100 = 19.29%
Check: 65.03 + 15.67 + 19.29 = 99.99
7. Calculate the percentage composition of sodium thiosulfate pentahydrate (Na2S2O3·5H2O), Atomic masses: Na: 22.990 g/mol, S: 32.066 g/mol, O: 15.999 g/mol, H: 1.008 g/mol
Answer
We have to account for the 5 molecules of water, therefore, for the calculations we need to calculate it as: (Na2S2O8H10)
Mr Na2S2O3·5H2O: 2 x 22.990 + 2 x 32.066 + 8 x 15.999 + 10 x 1.008 = 248.184 g/mol
% Na: (2 x 22.990 / 248.184) x 100 = 18.53%
% S: (2 x 32.066 / 248.184) x 100 = 25.84%
% O: (8 x 15.999 / 248.184) x 100 = 51.57%
% H: (10 x 1.008 / 248.184) x 100 = 4.06%
Check: 18.53 + 25.84 + 51.57 + 4.06 = 100
8. Calculate the percentage composition of lead acetate (Pb(C2H3O2)2). Atomic masses: Pb: 207.2 g/mol, C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol
Answer
Mr Pb(C2H3O2)2: 207.2 + 4 x 12.011 + 6 x 1.008 + 4 x 15.999 = 355.27 g/mol
% Pb: (207.2 / 355.27) x 100 = 58.32%
% C: (4 x 12.011 / 355.27) x 100 = 13.52%
% H: (6 x 1.008 / 355.27) x 100 = 1.13%
% O: (4 x 15.999 / 355.27) x 100 = 27.02%
Check: 58.32 + 13.52 + 1.13 + 27.02 = 99.99
9. Calculate the percentage composition of chloroform (CHCl3). Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, Cl: 35.453 g/mol
Answer
Mr CHCl3: 12.011 + 1.008 + 3 x 35.453 = 119.38 g/mol
% C: (12.011 / 119.38) x 100 = 10.06%
% H: (1.001 / 119.38) x 100 = 0.84%
% Cl: (3 X 35.453 / 119.38) x 100 = 89.09%
Check: 10.06 + 0.84 + 89.09 = 99.99
10. Calculate the percentage composition of glucose (C₆H₁₂O₆). Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol.
Answer
Mr C₆H₁₂O₆: 6 x 12.011 + 12 x 1.008 + 6 x 15.999 = 180.160 g/mol
% C: (6 x 12.011 / 180.160) x 100 = 40.00%
% H: (12 x 1.001 / 180.160) x 100 = 6.71%
% 0: (6 x 15.999 / 180.160) x 100 = 53.28%
Check: 40.00 + 6.71 + 53.28 = 99.99
Empirical formulas
1. Calculate the empirical formula of a compound which has a percent composition Mg: 20.19%, S: 26.64%, 0: 53.17%. Atomic masses: Mg: 24.305 g/mol, S: 32.066 g/mol, O: 15.999 g/mol
Answer
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| Mg | 24.305 | 20.19 | 20.19 / 24.305= 0.831 | 0.831 / 0831 = 1 |
| S | 32.066 | 26.64 | 26.64 / 32.066 = 0.831 | 0.831 / 0831 = 1 |
| O | 15.999 | 53.17 | 53.17 / 15.88 = 3.323 | 3.323 / 0831 = 4 |
Answer: MgSO4.
2. A sample of 100.00 g of iron oxide (FexOy) has 69.98 g of iron. What is its empirical formula? Atomic masses: Fe: 55.995 g/mol, O: 15.999 g/mol.
Answer
As the sample is 100 g and it has 69.98 g of iron, the rest is oxygen: 100 – 69.98 = 30.02 g of O
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| Fe | 55.995 | 69.98 | 69.98 / 55.995 = 1.251 | 1.251 / 1.251 = 1 |
| O | 15.999 | 30.02 | 30.02 / 15.99 = 1.876 | 1.876 / 1.251 = 1.50 |
To have whole numbers, we multiply by 2 and the answer is: Fe2O3.
3. A 2.000 g sample of uranium is heated in the air. The oxide formed (UxOy) formed weight 2.358 g. What is the empirical formula of the oxide? Atomic masses: U: 238.029 g/mol, O: 15.999 g/mol
Answer
The content of oxygen is 2.358 g – 2.000 g = 0.358 g
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| U | 238.029 | 2.000 | 2.000 / 238.029 = 0.008 | 0.008 / 0.008 = 1 |
| O | 15.999 | 0.358 | 0.358 / 15.999 = 0.022 | 0.022 / 0.008 = 2.66 |
Multiplying by 3, we get U3O8.
4. A compound is made of 13.260 g of Chromium, 12.265 g of sulfur and 24.475 g of oxygen. What is its empirical formula? Atomic masses: Cr: 51.996 g/mol, S: 32.066 g/mol and O: 15.999 g/mol.
Answer
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| Cr | 51.996 | 13.260 | 13.260 / 51.996 = 0.255 | 0.255 / 0.225 = 1 |
| S | 32.066 | 12.265 | 12.265 / 32.066 = 0.382 | 0.382 / 0.225 = 1.5 |
| O | 15.999 | 24.475 | 24.475 / 15.999 = 1.530 | 1.530 / 0.225 = 6 |
To have whole numbers, we multiply by 2: Cr2S3O12. This can be rearranged as Cr2(SO4)3, chromium sulfate.
5. Find the empirical formula of a compound that contains 43.38% of sodium, 11.33% of carbon and 45.29 of oxygen. Atomic masses: Na: 22.990 g/mol, C: 12.011 g/mol and O: 15.999 g/mol.
Answer
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| Na | 22.990 | 43.38 | 43.38 / 22.990 = 1.887 | 1.887 / 0.943 = 2 |
| C | 12.011 | 11.33 | 11.33 / 12.011 = 0.943 | 0.943 / 0.943 = 1 |
| O | 15.999 | 45.29 | 45.29 / 15.999 = 2.831 | 2.831 / 0.943 = 3 |
The compound is Na2CO3, sodium carbonate.
6. Find the empirical formula of a sample of 50.000 g that has the following composition: potassium 19.525 g, hydrogen: 0.505 g, carbon: 6.000 g and oxygen: 23.970 g. Atomic masses: K: 39.098 g/mol, H: 1.008 g/mol, C: 12.011 g/mol and O: 15.999 g/mol.
Answer
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| K | 39.098 | 19.525 | 19.525 / 39.098 = 0.499 | 0.499 / 0.499 = 1 |
| H | 1.008 | 0.505 | 0.505 / 1.008 = 0.501 | 0.501 / 0.499 = 1 |
| C | 12.011 | 6.000 | 6.000 / 12.011 = 0.500 | 0.500 / 0.499 = 1 |
| O | 15.999 | 23.970 | 23.970 / 15.999 = 1.498 | 1.498 / 0.499 = 3 |
The sample is KHCO3, potassium bicarbonate.
7. The combustion of 150.000 g an organic sample formed by carbon (C), hydrogen (H) and oxygen (O) combusts with oxygen to produce 260.787 g of carbon dioxide (CO2) and 143.505 g of water (H2O). What is the empirical formula of the sample? Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol.
Answer
The non-adjusted combustion reaction of the compound is:
CxHyOz + O2 → CO2 + H2O
As you can see, the carbon and the hydrogen are only provided by the sample, whereas the oxygen is provided partly by the compound and partly by the oxygen present in the air stream used in the combustion. The only way to calculate the oxygen present in the formulation is by subtracting to the mass of the sample, the masses of C provided the CO2 and the oxygen provided by the H2O:
Mass of O = mass of sample – mass of C – mass of H
Let’s go by parts.
We start with the C:
-
-
-
- The molecular mass of CO2 is 1 x 12.011 + 2 x 15.999 = 44.009 g/mol.
- The atomic mass of the C is 12.011 g/mol.
- Therefore, the proportion in masses between CO2 and the C is:
-
-
Mass of CO2 / Mass of C = 44.009 / 12.011
-
-
-
- As the sample provides 260.787 g of CO2, we have the following equation:
-
-
(Mass of CO2 / Mass of C =) 44.009 / 12.011 = 260.787 / mass of carbon
-
-
-
- That leads to:
-
-
mass of carbon = 12.011 x 260.787 / 44.009 = 71.029 g
Now the hydrogen:
-
-
-
- The molecular mass of H2O is 2 x 1.008 + 15.999 = 18.015 g/mol.
- The atomic mass of the H is 1.008 g/mol and in each molecule of H2O we have 2 hydrogens, their contribution is 1.008 x 2 = 2.016
- Therefore, the proportion in masses between H2O and the H is:
-
-
mass H2O / mass of H = 18.015 / 2.016
-
-
-
- As the sample provides 143.505 g of H2O, we have the following equation:
-
-
(mass H2O / mass of H =) 18.015 / 2.016 = 143.505 / mass of H
-
-
-
- That leads to:
-
-
mass of H= 143.505 x 2.016 / 18.015 = 16.059 g.
This means that:
Mass of O = mass of sample – mass of C – mass of H =
= 150.000 – 71.029 – 16.059 =
= 62.912 g
With these numbers, we can proceed as before:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 71.029 | 71.029 / 12.011 = 5.914 | 5.914 / 3.932 = 1.50 |
| H | 1.008 | 16.095 | 16.095 / 1.008 = 15.967 | 15.967 / 3.932 = 4.06 |
| O | 15.999 | 62.912 | 62.912 / 15.999 = 3.932 | 3.932 / 3.932 = 1.00 |
We multiply by 2 to get whole numbers and the answer is: C3H8O2.
8. The combustion of 200.000 g an organic sample formed by carbon (C), hydrogen (H) and oxygen (O) combusts with oxygen to produce 382.114 g of carbon dioxide (CO2) and 234.626 g of water (H2O). What is the empirical formula of the sample? Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol.
Answer
The procedure is the same that seen in the previous exercise. The non-adjusted combustion reaction of the compound is:
CxHyOz + O2 → CO2 + H2O
To calculate the mass of oxygen, we subtract to the mass of the sample, the masses of C provided the CO2 and the oxygen provided by the H2O:
Mass of O = mass of sample – mass of C – mass of H
If we proceed like the previous exercise, we can find that
-
- As the sample provides 382.114 g of CO2, we have the following equation:
(Mass of CO2 / Mass of C =) 44.009 / 12.011 = 382.114 / mass of carbon
This means:
mass of carbon = 44.009 / 12.011 = 382.114 x 12.011 / 44.099 = 104.287 g
-
- As the sample provides 234.626 g of H2O, we have the following equation:
(mass H2O / mass of H =) 18.015 / 2.016 = 234.626 / mass of H
This means:
mass of H = 234.626 x 2.016 / 18.015 = 26.256 g
-
- And total mass of carbon is:
Mass of O = mass of sample – mass of C – mass of H =
= 200.000 – 104.287 – 26.256 =
= 69.457 g
With these numbers:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 104.287 | 104.287 / 12,011 = 8.683 | 8.6831 / 4.341 = 2.00 |
| H | 1.008 | 26.256 | 26.256 / 1.008 = 26.048 | 26.048 / 4.341 = 6.00 |
| O | 15.999 | 69.457 | 69.457 / 15.999 = 4.341 | 4.341 / 4.341 = 1.00 |
The answer is: C2H6O.
Molecular formulas
1. A sugar contains 40.00% carbon, 6.71% of hydrogen and 53.28% of oxygen. If the molecular mass of the compound is 150.130 g/mol, calculate its molecular formula. Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol.
Answer
We start by calculating the empirical formula:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 40.00 | 40.00 / 12.011 = 3.330 | 3.330 / 3.330 = 1 |
| H | 1.008 | 6.71 | 6.71 / 1.008 = 6.657 | 6.657 / 3.330 = 2 |
| O | 15.999 | 53.28 | 53.28 /15.999 = 3.330 | 3.330 / 3.330 = 1 |
The empirical formula is CH2O, its molecular mass is:
atomic mass of C + 2 x atomic mass of H + atomic mass of O =
= 12.011 + 2 x 1.008 + 15.999 =
= 30.026 g/mol
The molecular formula is (CH2O)n, with
n = 150.130 / 30.026 = 5
The molecular formula is: C5H10O5.
2. An hydrocarbon contains 85.63% of carbon and its molecular mass is 84.162 g/mol. What is its molecular formula? Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol.
Answer
The first step is to calculate the percentage of hydrogen.
100.00 – 85.63 = 14.37 % of hydrogen.
We then calculate the molecular formula:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 85.63 | 85.63 / 12.011 = 7.129 | 7.129 / 7.129 = 1 |
| H | 1.008 | 14.37 | 14.37 / 1.008 = 14.256 | 14.256 / 7.129 = 2 |
The molecular formula is CH2, its molecular mass is:
atomic mass of C + 2 x atomic mass of H =
= 12.011 + 2 x 1.008 =
= 14.027 g/mol
The molecular formula is (CH2)n, with
n = 84.162 / 14.027 = 6
The molecular formula is: C6H12.
3. White or chrysotile asbestos is made of magnesium (28.03%), silicone (21.60%), hydrogen (1.16%) and oxygen (49.21%) O. Its molar mass is 520.206 g/mol. What is its molecular formula? Atomic masses: Mg: 24.305 g/mol, Si: 28.086 g/mol, H: 1.008 g/mol and O: 15.999g/mol.
Answer
We start by calculating the empirical formula:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| Mg | 24.305 | 28.03 | 28.03 / 24.305 = 1.153 | 1.153 / 0.769 = 1.50 |
| Si | 28.086 | 21.60 | 21.60 / 28.086 = 0.769 | 0.769 / 0.769 = 1.00 |
| H | 1.008 | 1.16 | 1.16 / 1.008 = 1.151 | 1.151 / 0.769 = 1.50 |
| O | 15.999 | 49.21 | 48.21 / 15.999 = 3.076 | 3.076 / 0.769 = 4.00 |
To have whole numbers, we multiply by 2 and the empirical formula is Mg3Si2H3O8, its molecular mass is:
3 x atomic mass of Mg + 2 x atomic mass of Si + 3 x atomic mass of H + 8 x atomic mass of O =
= 3 x 24.305 + 2 x 28.086 + 3 x 1.008 + 8 x 15.999 =
= 260.103 g/mol
The molecular formula is (Mg3Si2H3O8)n, with
n = 260.103 / 520.206 = 2
The molecular formula is: Mg6Si4H6O16.
4. Yellow acridine yellow has a percent composition of carbon: 75.92%, hydrogen: 6.37% and nitrogen: 17.71% with a molar mass of about 237.306 g/mol. Determine its molecular formula. Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol and N: 14.007 g/mol.
Answer
Calculation of the empirical formula:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 75.92 | 75.92 / 12.011 = 6.321 | 6.321 / 1.264 = 5.00 |
| H | 1.008 | 6.37 | 6.37 / 1.008 = 6.319 | 6.321 / 1.264 = 5.00 |
| N | 14.007 | 17.71 | 17.71 / 14.007 = 1.264 | 1.264 / 1.264 = 1.00 |
The empirical formula is C5H5N with molecular mass:
5 x atomic mass of C + 5 x atomic mass of H + atomic mass of N =
= 5 x 12.011 + 5 x 1.008 +14.007 =
= 79.102 g/mol
The molecular formula is (C5H5N)n, with
n = 237.306 / 79.102 = 3
The molecular formula is: C15H15N3.
5. The combustion of 250.000 g an organic sample formed by carbon (C), hydrogen (H) and oxygen (O) combusts with oxygen to produce 568.298 g of carbon dioxide (CO2) and 232.632 g of water (H2O). What is the molecular formula of the sample if its atomic mass is 116.160 g/mol? Atomic masses: C: 12.011 g/mol, H: 1.008 g/mol, O: 15.999 g/mol.
Answer
The non-adjusted combustion reaction of the compound is:
CxHyOz + O2 → CO2 + H2O
As you can see, the carbon and the hydrogen are only provided by the sample, whereas the oxygen is provided partly by the compound and partly by the oxygen present in the air stream used in the combustion. The only way to calculate the oxygen present in the formulation is by subtracting to the mass of the sample, the masses of C provided the CO2 and the oxygen provided by the H2O:
Mass of O = mass of sample – mass of C – mass of H
Let’s go by parts.
We start with the C:
-
-
- The molecular mass of CO2 is 1 x 12.011 + 2 x 15.999 = 44.009 g/mol.
- The atomic mass of the C is 12.011 g/mol.
- Therefore, the proportion in masses between CO2 and the C is:
-
Mass of CO2 / mass of C = 44.099/12.011
-
-
- As the sample provides 568.298 g of CO2, we have the following equation:
-
(Mass of CO2 / mass of C =) 44.099/12.011 = 568.298 / mass of C
-
-
- That leads to:
-
mass of C = 468.298 x 12.011 / 44.099 = 155.101 g
Now the hydrogen:
-
- The molecular mass of H2O is 2 x 1.008 + 15.999 = 18.015 g/mol.
- The atomic mass of the H is 1.008 g/mol and in each molecule of H2O we have 2 hydrogens, their contribution is 1.008 x 2 = 2.016
- Therefore, the proportion in masses between H2O and the H is:
mass H2O / mass H = 18.015/ 2.016
-
- As the sample provides 232.632 g of H2O, we have the following equation:
(mass H2O / mass H =) 18.015/ 2.016 = 232.632 / mass of H
-
- That leads to:
mass of H = 232.632 x 2.015 / 18.015 = 26.033 g
This means that:
Mass of O = mass of sample – mass of C – mass of H =
= 250.000 – 155.101 – 26.033 =
= 68.866 g
With this numbers, we can proceed as before:
| Atom | Atomic mass | Mass (or %) | Number of moles (mass/atomic mass) | Number of moles/lowest number of moles |
| C | 12.011 | 155.101 | 155.101 / 12.011 = 12.913 | 12.913 / 4.304 = 3 |
| H | 1.008 | 26.033 | 26.033 / 1.008 = 25.826 | 25.826 / 4.304 = 6 |
| O | 15.999 | 68.866 | 68.866 / 15.999 = 4.304 | 4.304 / 4.304 = 1 |
Therefore, the empirical formula is C3H6O, its molecular mass is:
3 x atomic mass of C + 6 x atomic mass of H + atomic mass of O =
= 3 x 12.011 + 6 x 1.008 + 15.999=
= 58.080 g/mol
The molecular formula is (C3H6O)n, with
n = 116.160 / 58.080 = 2
The molecular formula is: C6H12O2.
Comments
If you have any question, or want to add anything else, please leave a comment below.
